By Teresa Lara-Meloy | February 8, 2019
I recently started a martial arts class. Towards the end of the first class, we began something I have never done before: the five animal poses. As I focused on following the leader, making sure I’ve got the right motions, we embodied different animals, starting with the bear. I could see the hands for these movements were always in claw-like shape. That made sense. The next animal was the tiger. Suddenly, the class started growling and roaring. Because I was totally clueless as to what was expected of me, I started to panic. Is that how we were to embody the tiger? In searching for clues, I looked around and saw that not everyone was engaging in these vocalizations, even when they were all following the stealthier, cat-like version of the poses. I felt some comfort in knowing I was not alone and continued with the class. We proceeded through the rest of the animals, ending with the deer – which is apparently a more silent animal. I sighed in relief.

What does this story have to do with math argumentation, you may ask. Think about what is usually expected of students during math class. In the past, and even many classrooms today, a math class involved the teacher presenting a lesson, then students practicing the procedures therein, and the teacher correcting students along the way. But things are changing!
Now, we ask students to participate in math conversations, and in particular, we ask them to engage in mathematical argumentation. In this new situation, students may not always know what to do, what is expected of them, and what successful participation in math class means. In fact, I suspect that their level of panic when confronted with new ways of engaging in math discussions and class is similar (or possibly worse) than what I felt in that martial arts class.

In Mathematical Argumentation in Middle School – The What, Why, and How, we propose the use of warm up games to help students learn productive norms for classroom discussion and each stage of argumentation (See our suggested norms for argumentation). These games are particularly important to play at the beginning of the year, to get the class off to the right start. But we suggest using these games throughout the year. They are a great way to open class, using a playful, non-mathematical way to remind students of how to be respectful, listen to one another, make bold conjectures, build off of other people’s ideas, and be unafraid to make mistakes. Each game has simple rules of interaction that foster spontaneity and strong collaboration among participants. The games bridge between students’ everyday experiences and the academic practice of mathematics in a lively, engaging way that is safe even for those with little confidence in their mathematical competence. Once you play a game, it is important to make explicit, through discussions, what the connection is between the norms and the game.
While there are games for each of the stages of argumentation in our book, here’s one game to help students see that building an argument in class is like telling a story together. In the game, students learn that it is important to listen to others (before you), so that you can easily make a contribution when it is your turn. Then you, as their math teacher, connect that idea to math conversations. The games are non-mathematical in nature, to make them accessible to all students, even those with less confidence in math.

See our book for more games and ideas of how to establish fun and explicit classroom norms for engaging your students in argumentation.
This blog post was originally published on Corwin Connect: http://corwin-connect.com/2018/11/establishing-norms-for-mathematical-argumentation/
Sources:
- Tiger image: https://www.publicdomainpictures.net/en/view-image.php?image=26472&picture=chinese-style-tiger-art. License: CC0 Public Domain
- How to Do Math Argumentation poster
- Mathematical Argumentation in Middle School—The What, Why, and How by Jennifer Knudsen, Harriette S. Stevens, Teresa Lara-Meloy, Hee-Joon Kim, and Nicole Shechtman. Thousand Oaks, CA: Corwin: page 130.
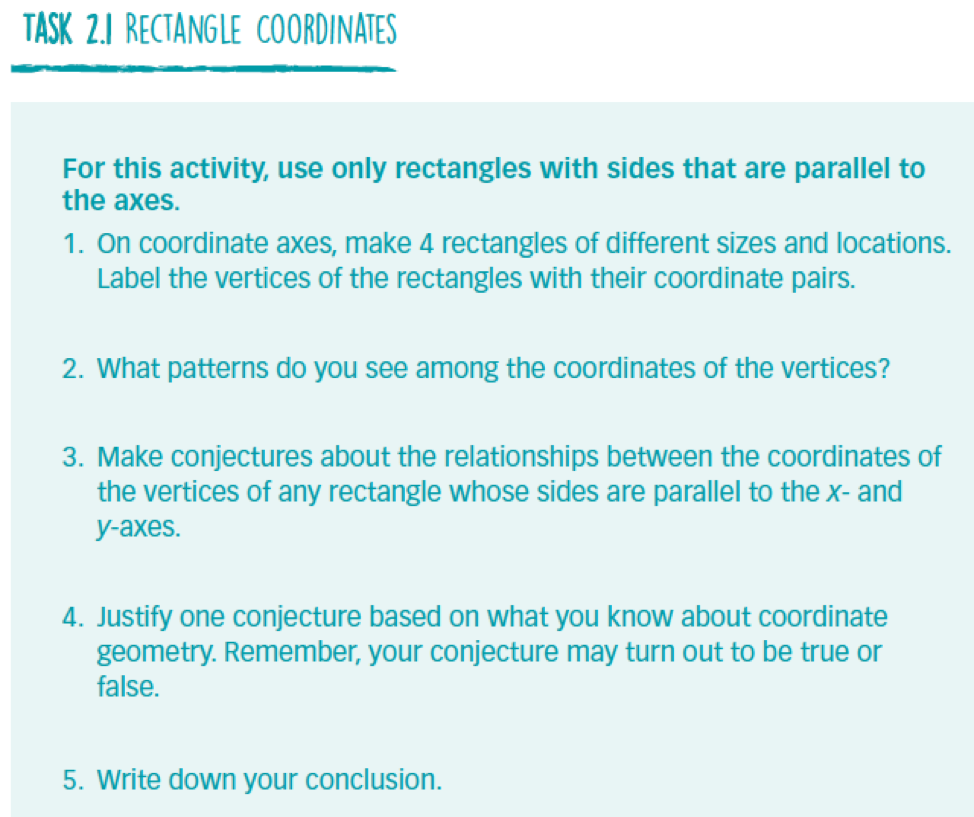
 A simple, particular conjecture could be a guess at what value of x makes 3x+35 =74 true. That would be a very particular conjecture. Students could approach it, before they know how to solve “two step equations,” by making their best guess. What if x is 10?
A simple, particular conjecture could be a guess at what value of x makes 3x+35 =74 true. That would be a very particular conjecture. Students could approach it, before they know how to solve “two step equations,” by making their best guess. What if x is 10?